The geometry of time
Fourth dimension concept
"It is absolutely possible that beyond what our senses perceive, hide unsuspected worlds." Albert Einstein (1879 - 1955), Nobel Prize in Physics in 1921 for his interpretation of the photoelectric effect.
In 1905, Albert Einstein in his formulation of special relativity, told us that space and time are linked in a four-dimensional continuum. Objects move in a space-time where the coordinate of time is no longer differentiated from the three coordinates of space.
Time has become a dimension of space, that is to say an additional direction according to which an object can move.
How to represent this fourth dimension?
A zero dimensional space is a space in which we move in zero direction, that is, we cannot move, it is a point. If we stretch a zero dimensional space we get a one dimensional space, that is to say a line. An object can only move in one direction but in both directions. The direction chosen here is horizontal but we could have represented it from another point of view (oblique direction). If we stretch a one-dimensional space we get a two-dimensional space, that is to say a plane. In a plane, two directions are enough for an object to move anywhere. The directions chosen here (the simplest) are orthonormal but we could have taken two oblique directions. If we stretch a two-dimensional space we get a three-dimensional space, that is to say a volume. Three directions are enough for an object to move anywhere in the volume. The directions chosen here (the simplest) are perpendicular to each other but we could have taken three oblique directions. If we stretch a three-dimensional space, we obtain a four-dimensional space, that is to say a hypervolume. Four directions are enough for an object to move anywhere in the hypervolume. The directions chosen here (the simplest) are perpendicular to each other but we could have taken oblique directions.
The definition of a four-dimensional space-time where the notion of time disappears is easy to understand. However, the rigorous representation of this space in 4D is extremely complex or even impossible to image.
Our senses have not been selected by evolution to see the universe in infrared or ultraviolet. They were also not selected to perceive more than three dimensions. Moreover our universe is projected in two dimensions on our retina (as on a screen). But by moving in this three-dimensional space (changing direction, turning, etc.), our brain accumulates more and more 2D viewpoints and can interpret a third dimension (depth).
Yet we also move in time!
This faculty of the brain which allows us to see in 3D has been a selective advantage and has therefore been selected by evolution. But the interpretation of 4D has not been a selective advantage, and our movement from the past to the future has not been integrated with the other three directions.
It is therefore impossible to imagine this fourth dimension. However, it is possible to mentally represent one or more partial images of reality.
These images cannot be reality itself because our brain has no experience of 4D unlike 3D in which we operate every day.
NB: the notion of space-time was introduced by Minkowski in 1908 in a mathematical exposition on the geometry of space and time as it had been defined by the special theory of relativity of Albert Einstein "On the electrodynamics of moving bodies".
The formulation of special relativity that Minkowski proposed in 1908 had perhaps even more difficulty in asserting itself than Einstein's initial formulation. Indeed, Einstein himself began by pushing her away... The reason is that, as Minkowki had announced, time and space, which Einstein had already made relative, received the status of illusions and disappeared to make way for space-time, a concept that was too mathematical. and abstract to Einstein's taste. But as he himself admitted, Einstein could never have formulated his theory of general relativity without the concept of space-time formalized by Minkowski.
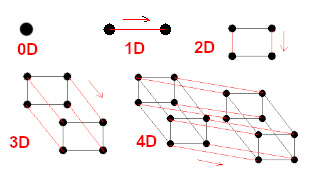
Image: A zero dimensional space is a point. If we stretch the point, we get a one-dimensional space, i.e. a line. If we stretch the line, we get a two-dimensional space, that is to say a plane. If we stretch the plane, we get a three-dimensional space, that is to say a volume. If we stretch the volume, we get a four-dimensional space, that is to say a hypervolume.
In this representation, each new dimension is perpendicular to the previous ones up to the 4D hypercube, but we can continue in this way in dimension five, then six, and with n-dimensional or n-cube hypercubes. The representation of the hypercube is the simplest, but it is the same for a hypersphere, a hyperprism or a non-geometric hypervolume.
An object evolving in dimension n cannot see another object evolving in dimension n + 1. For example, in 2D space, no 3D object is visible.

Image: The four-dimensional or 4-cube hypercube is often referred to as "tesseract".
Partial images in space-time
Each time we add a dimension to the mathematical space, it grows enormously.
The representation of a four-dimensional universe is much larger than the three-dimensional universe. In 2D space a circle is a slice of the sphere in 3D space.
Likewise, in our familiar three-dimensional universe one can only see a single slice of the four-dimensional universe.
To try to illustrate this four-dimensional state, we must reduce the number of dimensions.
A two-dimensional space-time (one dimension of space and one dimension of time) suffices to represent the concept of the geometry of time.
The space dimension can only contain points or lines that can only move along this dimension. In two-dimensional space-time, imagine a figure as in the image opposite.
A slit will move in the dimension of time, from the past to the future (from bottom to top). The view of our 2D universe through the slit shows us successive points and lines. The noticeable part of the 2D universe is what appears in the slit. As the slit moves from bottom to top, as time goes by, the dots and lines move horizontally along the slit. In the same way in a 3D universe what appears in a 2D universe is what appears in the slit. And so on...
Thus, we only perceive a three-dimensional slice of a four-dimensional reality. In our familiar 3D universe, time would only be a dynamic way of seeing the much larger 4D static universe in which the past, present and future do not exist. Everything would be frozen and visible in one fell swoop by beings from the fourth dimension !!!
NB: "Time is the best way to find nature so that everything does not happen all at once." Etienne Klein in the video "Does time exist?"
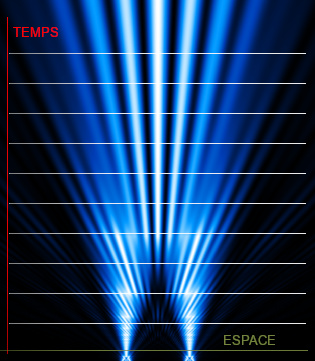
Image: representation of an image in two-dimensional space. A single slit slides on the figure from bottom to top representing the passage of time. What is perceptible is what appears in the slit of 1D space. We do not see the whole of the 2D figure but the figure slices after slice, that is to say small parts which scroll one after the other. The space seen by the slit seems dynamic, everything moves, while the 2D space is frozen. The illusion of dynamics is caused by the slippage of the slit, that is, by the passage of time.
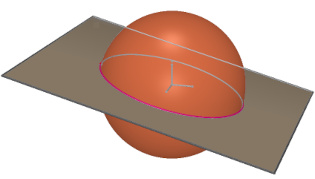
Image: A sphere crossing a two-dimensional universe would appear seen from that universe as a circle (red line) increasing more and more until the equator of the sphere then decreasing until disappearing.
The 2D world can only see the edge of the sphere which is inside its plane, that is to say a circle. 2D physical space is just a slice of 3D space. What the world sees in 2D is what appears on the map. The top and bottom are invisible.
Likewise, our familiar 3D physical space is actually just a 3D slice of a real 4D space that encompasses it.
If a 4D space hypersphere crossed our 3D space, we would see the layers of the hypersphere appear successively. We would see a small sphere which would grow little by little until it reached the size of the equator of the hypersphere then which would decrease until it completely disappeared. We would have seen the hypersphere slice by slice.
Articles on the same theme
1997 © Astronoo.com − Astronomy, Astrophysics, Evolution and Ecology.
"The data available on this site may be used provided that the source is duly acknowledged."
How Google uses data
Legal mentions
English Sitemap − Full Sitemap
Contact the author
 Water Anomalies: A Common and Abundant Molecule in the Universe
Water Anomalies: A Common and Abundant Molecule in the Universe  What is Dust? Between the Dust on Our Shelves and the Dust that Builds Planets
What is Dust? Between the Dust on Our Shelves and the Dust that Builds Planets  Heat and Temperature: Two Often Confused Thermal Notions
Heat and Temperature: Two Often Confused Thermal Notions  Electroweak Force: The Unification of Electromagnetism and the Weak Interaction
Electroweak Force: The Unification of Electromagnetism and the Weak Interaction  Special Relativity: The Beginning of a New Physics
Special Relativity: The Beginning of a New Physics  The Higgs Boson: The Unification of Fundamental Forces
The Higgs Boson: The Unification of Fundamental Forces Quantum Entanglement: When Two Particles Become One!
Quantum Entanglement: When Two Particles Become One! The Pentaquark: A New Piece of the Cosmic Puzzle!
The Pentaquark: A New Piece of the Cosmic Puzzle! Why are Rare Gases
rare?
Why are Rare Gases
rare? Brownian Motion: A Link Between Two Worlds
Brownian Motion: A Link Between Two Worlds The 4 Articles of Albert Einstein from 1905
The 4 Articles of Albert Einstein from 1905  Why does nuclear fusion require so much energy?
Why does nuclear fusion require so much energy?  Feynman diagrams and particle physics
Feynman diagrams and particle physics  Stars cannot create elements heavier than iron because of the nuclear instability barrier
Stars cannot create elements heavier than iron because of the nuclear instability barrier  Alpha, Beta, and Gamma Radiation: Understanding Their Differences
Alpha, Beta, and Gamma Radiation: Understanding Their Differences  Planck wall
theory
Planck wall
theory  Is
emptiness really empty?
Is
emptiness really empty?  Giant Colliders: Why the LHC is Unique in the World
Giant Colliders: Why the LHC is Unique in the World  The World of Hadrons: From the LHC to Neutron Stars
The World of Hadrons: From the LHC to Neutron Stars  Radioactivity, natural and artificial
Radioactivity, natural and artificial  The World of Nanoparticles: An Invisible Revolution
The World of Nanoparticles: An Invisible Revolution  Schrodinger's Cat
Schrodinger's Cat
 Before
the big bang the multiverse
Before
the big bang the multiverse  Eternal
inflation
Eternal
inflation  What
is a wave?
What
is a wave?  The fields of reality: what is a field?
The fields of reality: what is a field?  Space in time
Space in time
 Quantum computers
Quantum computers
 Bose-Einstein condensate
Bose-Einstein condensate 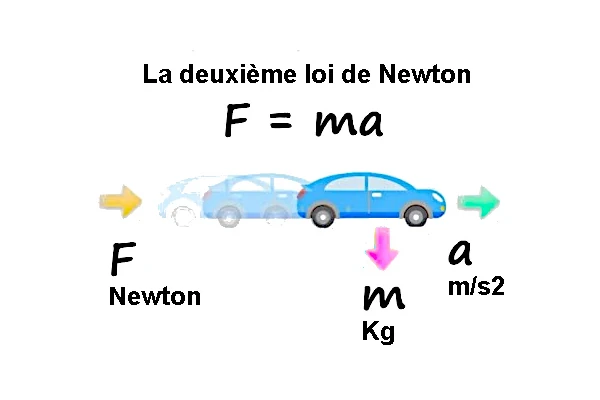 Equation of Newton's three laws
Equation of Newton's three laws  Field concept
in physics
Field concept
in physics  The electron, a kind of electrical point
The electron, a kind of electrical point  Entropy and
disorder
Entropy and
disorder  The
infernal journey of the photon
The
infernal journey of the photon  Mystery of the Big Bang, the problem of the horizon
Mystery of the Big Bang, the problem of the horizon  Beta Radioactivity and Neutrino: A Story of Mass and Spin
Beta Radioactivity and Neutrino: A Story of Mass and Spin  Spacetime: Space and Time United, understand this concept
Spacetime: Space and Time United, understand this concept  Time Measurement: Scientific and Technological Challenge
Time Measurement: Scientific and Technological Challenge  Physical and Cosmological Constants: Universal Numbers at the Origin of Everything
Physical and Cosmological Constants: Universal Numbers at the Origin of Everything  Spectroscopy, an inexhaustible source of information
Spectroscopy, an inexhaustible source of information  Abundance of chemical elements in the universe
Abundance of chemical elements in the universe  The size of atoms
The size of atoms
 Magnetism and Magnetization: Why Are Some Materials Magnetic?
Magnetism and Magnetization: Why Are Some Materials Magnetic?  The quark confinement
The quark confinement
 Superpositions of quantum states
Superpositions of quantum states  Alpha decay (α)
Alpha decay (α)
 Electromagnetic induction equation
Electromagnetic induction equation  Fusion and Fission: Two Nuclear Reactions, Two Energy Paths
Fusion and Fission: Two Nuclear Reactions, Two Energy Paths  From the Ancient Atom to the Modern Atom: An Exploration of Atomic Models
From the Ancient Atom to the Modern Atom: An Exploration of Atomic Models  The Origins of Mass: Between Inertia and Gravitation
The Origins of Mass: Between Inertia and Gravitation  From the Nucleus to Electricity: Anatomy of a Nuclear Power Plant
From the Nucleus to Electricity: Anatomy of a Nuclear Power Plant  The Universe
of X-rays
The Universe
of X-rays  How
many photons to heat a coffee?
How
many photons to heat a coffee?  Seeing Atoms: An Exploration of Atomic Structure
Seeing Atoms: An Exploration of Atomic Structure  Quantum tunneling of quantum mechanics
Quantum tunneling of quantum mechanics  Entropy: What is Time?
Entropy: What is Time?  The 12 Particles of Matter: Understanding the Universe at the Subatomic Scale
The 12 Particles of Matter: Understanding the Universe at the Subatomic Scale  The Atomic Orbital: Image of the Atom
The Atomic Orbital: Image of the Atom  Antimatter and antiparticle
Antimatter and antiparticle  What is an
electric charge?
What is an
electric charge?  Our matter
is not quantum!
Our matter
is not quantum!  Why use hydrogen in the fuel cell?
Why use hydrogen in the fuel cell?  Newton and Einstein: Two Visions for the Same Mystery
Newton and Einstein: Two Visions for the Same Mystery  Where does the proton's mass come from?
Where does the proton's mass come from?  Einstein's Universe: Physical Foundations of the Theory of Relativistic Gravitation
Einstein's Universe: Physical Foundations of the Theory of Relativistic Gravitation  1905, The Silent Revolution: When Einstein Rewrote the Laws of Nature
1905, The Silent Revolution: When Einstein Rewrote the Laws of Nature  What does the equation E=mc2 really mean?
What does the equation E=mc2 really mean?  Between Waves and Particles: The Mystery of Duality
Between Waves and Particles: The Mystery of Duality  The Supercritical State of Water: Between Liquid and Gas, a Fourth Phase?
The Supercritical State of Water: Between Liquid and Gas, a Fourth Phase?  Quantum Mechanics and Spirituality: Another Way to See the World
Quantum Mechanics and Spirituality: Another Way to See the World