Description of the image: Warren McCulloch (1898-1969) and Walter Pitts (1923-1969) introduced what they called "formal neurons." These formal neurons are a mathematical abstraction of biological neurons, designed to represent their functioning in a simplified yet sufficiently precise manner to allow for mathematical analysis.
McCulloch, a neurophysiologist, and Pitts, a logician, conceptualized formal neurons as computational units to construct artificial neural networks in their 1943 paper titled "A Logical Calculus of the Ideas Immanent in Nervous Activity."
In this publication, they presented a simple yet powerful mathematical model of neurons and neural networks. Their objective was to understand how the brain could perform logical operations, similar to those performed by a computer.
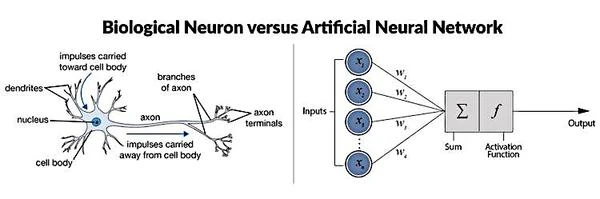
A formal neuron is a mathematical and computational representation of a biological neuron. It has multiple inputs and one output, corresponding to the dendrites and the axon hillock of the biological neuron, the starting point of the axon. The excitatory and inhibitory actions of the synapses are represented by numerical coefficients (synaptic weights) associated with the inputs.
Within their model, a formal neuron takes binary inputs (activated or deactivated) from other neurons or external sources. Each input is weighted by a specific weight, which can represent the strength of the synaptic connection between neurons in the biological model.
Based on the weighted sum of its inputs, the formal neuron produces a binary output, which can be activated (1) or deactivated (0). This output is usually determined by applying an activation function, such as the threshold function, which converts the weighted sum of inputs into a binary output according to a predefined threshold.
McCulloch and Pitts used these formal neurons to construct artificial neural networks capable of performing logical operations such as conjunction (AND), disjunction (OR), and negation (NOT). They demonstrated that even with these simple connection rules, it was possible to build neural networks capable of performing complex logical operations.
This formalization of neurons was crucial for enabling mathematical analysis and modeling of neural networks, thus laying the foundation for future research in artificial intelligence and computational neuroscience (the application of computer science to the understanding of the nervous system).
The First Step Towards the Emergence of Life 
Phylogenetic Tree of Life 
The
shadow biosphere 
Decline of Anthropocentrism 
Artificial intelligence: the explosion of gigantism 
When AI models train on their own data, they go mad! 
Emergence of artificial intelligence: Illusion of intelligence or intelligence? 
The
horseshoe crab, a living fossil! 
Biosignatures or presence of life in the Universe 
Challenge and threat of Artificial Intelligence 
How do machines understand, interpret and generate language in a similar way to humans? 
How does an artificial neural network work? 
Origin of life on Earth: Panspermia theory 
Origin of life on Earth: White smoker theory 
Why 37 degrees Celsius? 
Thermodynamics of the sandpile 
Are we alone in the universe? 
Trace of frozen life in Siberia 
Ice cores tell us about our past 
Life evolves in the shelter of glaciations 
Organ regeneration, the salamander 
Cosmic rays and the mutation of species 
Mephisto, the little worm of the depths 
Discovery of solid buckyballs in space 
Bipedalism in
hominids 
Kamchatka
giant crab 
The passage between the inert and the living 
From particles to biochemical life 
Egocentric vision, the man at the center 
Megapod
uses volcanic heat 
Ardi is 4.4 million years old 
Natural selection, the birch moth 
The explosion of life in the Ordovician 
Liquid water, an accelerator of chemical reactions 
Neandertal 
Asimo the
future humanoid 
Conditions for the appearance of life 
Fermi's paradox or Plato's cave 
The Tardigrade, the immortal animal 
Toumaï,
7 million years old 
Border between inanimate and living 
The incredible life of the abyss 
Cyanobacteria create toxic gas 
The short history of the evolution of life 
The smallest frog in the world 
The explanation of the Little Ice Age 
Ashen light, the proofs of life 
Bioluminescence
of living organisms 
Beyond our senses, the great scientific revolutions 
The primitive
soup