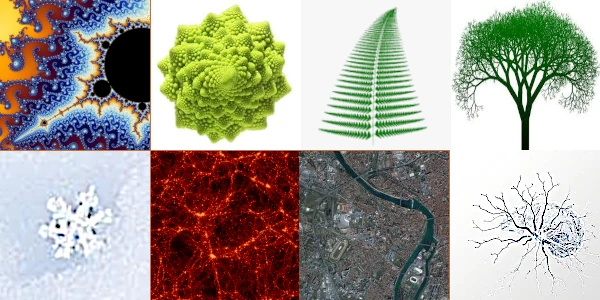
A fractal is a geometric structure possessing the property of self-similarity: each portion, regardless of the scale of observation, resembles the whole. This property finds a striking expression in mountain reliefs, river branches, pulmonary and neural networks, and even in the distribution of galaxies in the observable universe.
The fractal imprint is omnipresent in nature. Fractals are complex, self-similar geometric structures found everywhere in nature. Their pattern repeats at different scales, creating both irregular and harmonious shapes.
Fractals reveal a fundamental principle of nature: the ability to generate complexity from simple rules. These natural structures share an essential property: self-similarity or the recurrence of patterns at different scales. This emergence of order from chaos is a visible manifestation of complex physical processes self-organizing.
These natural forms show that fractals are not just abstract: they are fundamental in the organization of the living and physical world.
| Structure | Description | Scale | Type of Fractality |
|---|---|---|---|
| Galaxies | Spiral arms and clusters show a fractal organization on a large scale, up to 500 Mpc. | 10⁴ to 10⁸ light-years | Statistical (hierarchical distribution) |
| Cloud Structures | Self-similar contours, influenced by atmospheric turbulence. | 10 m to 100 km | Physical (turbulence, energy cascade) |
| Blood Flow | Branched network of arteries and capillaries optimizing diffusion. | µm to m | Biological (optimization network) |
| Neural Networks | Dendritic branches maximizing connections and information processing. | µm to cm | Biological and Functional |
| Breton Coast | Irregular geometry revealing an increasing length as you zoom in. | 1 to 1000 km | Geomorphological |
| Lungs | Branching of the bronchi to the alveoli: 23 fractal generations. | mm to 30 cm | Biological (maximal surface/volume) |
| Bacterial Colonies | Growth in dendritic patterns on poor substrates. | mm to cm | Diffusion-limitation |
| Mountains and Reliefs | Persistent roughness over several orders of magnitude. | 10 m to 1000 km | Geophysical (erosion, deposition, tectonics) |
| Snowflakes | Hexagonal dendritic crystallization sensitive to temperature and humidity. | 0.1 to 10 mm | Physical (growth instabilities) |
| Spider Webs | Regular spiral patterns with geometric recurrence. | cm to tens of cm | Functional Bioconstruction |
| Romanesco Broccoli | Pyramidal spirals following the Fibonacci sequence. | cm to dm | Visible Vegetal Fractal |
| Ammonites | Fossil shells in equiangular logarithmic spiral. | cm to tens of cm | Natural Geometric |
| Other Examples | Ferns, lightning, river networks, corals, urban formations. | µm to km | Multidisciplinary |
1997 © Astronoo.com − Astronomy, Astrophysics, Evolution and Ecology.
"The data available on this site may be used provided that the source is duly acknowledged."
How Google uses data
Legal mentions
English Sitemap − Full Sitemap
Contact the author
Major Civilizational Collapses: Key Periods and Causes
Generative AI vs AGI: Where does imitation end and consciousness begin?
Declining Births: Demographic Catastrophe or Natural Evolution? 
Natural Selection vs. Chance: Why Evolution is Not a Lottery? 
What if Life Originated from Earth? A Revolution in the Theory of Panspermia 
The Great Bifurcation that will Disrupt Our World: Survival or Collapse? 
Primordial Chemistry: Where Do the First Organic Molecules Originate? 
CO and CO₂: Two Gases, Two Risks, Two Biological Mechanisms 
Spontaneous Synchronization: A Universal Phenomenon, from Physics to Life 
Artificial networks vs biological networks: Two systems, one common architecture 
Human Brain and Artificial Intelligences: Similarities and Differences 
Time Challenge: How to Illustrate a Billion Years? 
The Three Essential Components for the Emergence of Life 
Why Did the Genus Homo Nearly Go Extinct 900,000 Years Ago? 
AlphaGo vs AlphaGo Zero: A Revolution in Artificial Intelligence 
The Next Step for Intelligent Machines 
The First Step Towards the Emergence of Life 
Phylogenetic Tree of Life 
Formal neuron 
The shadow biosphere 
Decline of Anthropocentrism 
Artificial intelligence: the explosion of gigantism 
When AI models train on their own data, they go mad! 
Emergence of artificial intelligence: Illusion of intelligence or intelligence? 
The horseshoe crab, a living fossil! 
Biosignatures or presence of life in the Universe 
Challenge and threat of Artificial Intelligence 
How do machines understand, interpret and generate language in a similar way to humans?
How does an artificial neural network work? 
Origin of life on Earth: Panspermia theory 
Origin of life on Earth: White smoker theory 
Why 37 degrees Celsius? 
Thermodynamics of the sandpile 
Are We Alone in the Cosmos? Between Science and Speculation 
Trace of frozen life in Siberia 
Ice cores tell us about our past 
Life evolves in the shelter of glaciations 
Organ regeneration, the salamander 
At the Limits of Life: Mephisto, Worm of the Infernal Depths 
Discovery of solid buckyballs in space 
Human Walking: The Origins of Bipedalism in Hominids 
Kamchatka giant crab 
The passage between the inert and the living 
From particles to biochemical life 
Sediba: Man or Australopithecus 
Egocentric vision, the man at the center 
Megapod uses volcanic heat 
Ardi is 4.4 million years old 
Natural selection, the birch moth 
The explosion of life in the Ordovician 
Liquid Water, Much More Than a Solvent: A Catalyst for Chemical Reactions 
Neanderthal: Humanity's Lost Cousin 
Asimo the future humanoid 
What Conditions Allowed the Emergence of Life? 
Fermi's paradox or Plato's cave 
The Tardigrade, the immortal animal 
Toumaï, 7 million years old 
Border between inanimate and living 
The incredible life of the abyss 
Cyanobacteria and the Oxygen Crisis: A Primordial Ecological Catastrophe 
From Matter to Life: The Blurred Frontier of Biological Emergence 
The Smallest Frog in the World: Physiological Secrets of a Microvertebrate 
The explanation of the Little Ice Age 
The Light of Life: A Biosignature Revealed by the Moon 
Living Light: The Dazzling Secrets of Bioluminescence 
Beyond our senses, the great scientific revolutions 
The primitive soup 
World Population: From One Billion Humans to Demographic Saturation 
Ecology and Collapse: The Case of Easter Island 
Fractals: Universally Self-Organized Structures