Description of the image: The fundamental ideas about mechanical energy and the conservation of energy were gradually established with the contributions of many scientists. The unit of measurement for mechanical energy in the International System (SI) is the joule, symbol J.
The concept of energy, as we understand it today, has evolved over the centuries. It was long confused with force and power.
The word 'energy' first appeared in 1717 in a letter written by Jean Bernoulli (1667-1748) to define mechanical work. However, it was not until the mid-19th century that the principle of conservation of energy was established. Max Planck (1858-1947) understood the essential scope of this law in his 1887 book, "The Principle of Conservation of Energy".
The central idea is that energy is a constant in the universe, that it cannot be created or destroyed, but that it takes on different forms over time.
The second essential equation in physics, often taught just after Newton's second law, is the equation of the conservation of mechanical energy in an isolated system.
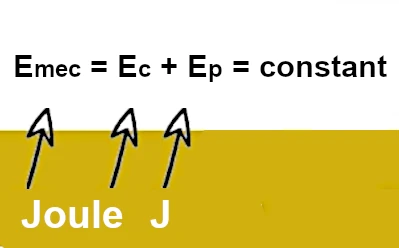
Eméc = Ec + Ep = constant
• Eméc is the total mechanical energy of the system in Joules.
• Ec = ½ mv2 is the kinetic energy, where m is the mass of the object and v is its velocity. Contributions from Jean le Rond d'Alembert (1717-1783) and other scientists, such as Daniel Bernoulli (1700-1782), were crucial for the development of this formula.
• Ep = mgh is the potential energy, which depends on the nature of the force field considered (gravitational, elastic, etc.), where h is the height relative to a reference point and g is the acceleration due to gravity (9.80665 m/s²). This formula is associated with the work of Isaac Newton (1643-1727).
Potential energy can be converted into kinetic energy and vice versa. For example, a falling object transforms its gravitational potential energy (mgh) into kinetic energy (½ mv2). Since energy is conserved, Ec + Ep = constant.
The joule was chosen to measure the various types of energy.
This equation is crucial because it expresses the principle of conservation of energy, one of the most fundamental principles of physics. It shows that, in an isolated system (without exchange of energy with the outside), the total energy remains constant, even if it can be transformed from one form to another (for example, from potential energy to kinetic energy and vice versa).
James Prescott Joule (1818-1889) played a crucial role in establishing this principle with his experiments on the conservation of thermal and mechanical energy.
To measure an amount of energy, the joule is the most appropriate unit. It provides a "snapshot" of the energy present in a system. For example, the energy stored by a dam due to its position in the gravitational field (Ep = mgh) is a quantity of energy even though the water is at rest.
By combining information on mass, height, and acceleration due to gravity, we can calculate the stored gravitational potential energy. This energy represents the potential of the water to do work (such as turning a turbine) when it falls under the effect of gravity.
Using the Joule helps unify the measurement of different forms of energy and simplify calculations in physics.
The watt, which is a very common unit, especially in electricity, does not measure the same thing as the joule.
It is the unit of power.
Power is the rate at which energy is transferred or transformed. In other words, the watt measures the amount of energy per unit of time. For example, 1 kilowatt-hour.
Energy is a quantity; power is a rate.
For example, in a water pipe, the flow rate of water (in liters per second) corresponds to power, while the total amount of water coming out of the pipe (in liters) corresponds to energy.
1 watt is equivalent to 1 joule per second. A 100-watt light bulb consumes 100 joules of electrical energy every second.
The Third Essential Equation in Physics
The first essential equation in physics
The electromagnetic force or Lorentz force
The solar energy received depends on the angle of incidence
Why is marble colder than wood?
Planck's wall theory